To add fractions, first convert them to fractions with common denominators. (See Comparing apples to apples.)

Here's an example of adding fractions. Follow the steps below to add the fractions 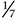 and
and 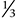 .
.
Find the lowest common denominator. Because the denominators in
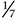 and
and 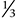 are both prime numbers, multiply 7 by 3 to find the lowest common denominator, 21.
are both prime numbers, multiply 7 by 3 to find the lowest common denominator, 21.Then convert the fractions by multiplying each by 1 (in the form of a fraction) to yield fractions with the lowest common denominator.
Start by converting
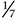 to
to 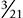 :
:
Then convert
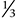 to
to 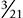
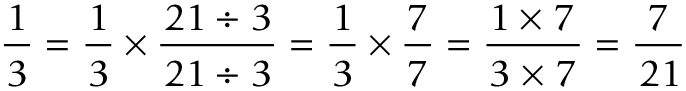
Now, add the new fractions. To add fractions with a common denominator, add the numerators and place the result over the common denominator. The resulting fraction is your answer. (Reduce it to its lowest terms, if possible.)
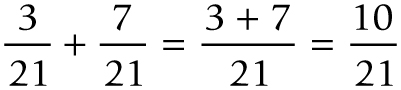
To add 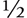 and
and 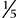 , follow these steps:
, follow these steps:

Find the lowest common denominator. In this case, because the denominators 2 and 5 are both prime numbers, multiply 2 by 5 to find the lowest common denominator, 10.
Convert the fractions by multiplying each by 1 (in the form of a fraction) to yield fractions with the lowest common denominator.
Convert the fraction
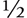 to
to 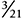 :
:
Then convert
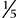 to
to 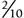 :
: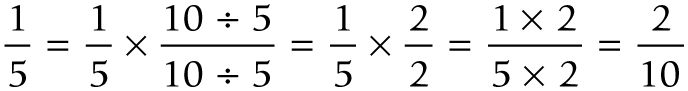
Now, add the converted fractions. To do this, add the numerators and place the result over the common denominator:
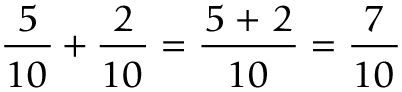

To add 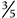 and
and 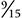 , follow these steps:
, follow these steps:
Find the lowest common denominator—in this case, 15.
Convert the fractions by multiplying each by 1 (in the form of a fraction) to yield fractions with the lowest common denominator.
Convert to
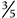 to
to 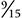 :
: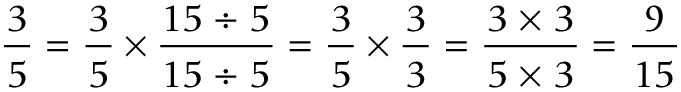
Then convert
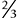 to
to 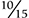 :
: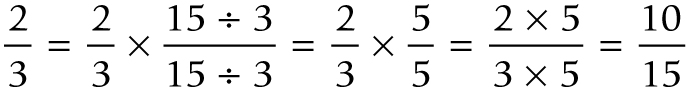
To add the converted fractions, add the new numerators and place the result over the common denominator:

Reduce the fraction to its lowest terms:
